Properties of Matter waves
All matter can exhibit wave-like behaviour. For example, a beam of electrons can be diffracted just like a beam of light or a water wave. Matter waves are a central part of the theory of quantum mechanics, an example of wave-particle duality. The concept that matter behaves like a wave is also referred to as the de Broglie hypothesis (/dəˈbrɔɪ/) due to having been proposed by Louis de Broglie in 1924. Matter waves are often referred to as de Broglie waves.
The de Broglie wavelength is the wavelength, λ, associated with a particle and is related to its momentum, p, through the Planck constant, h:
Wave-like behaviour of matter was first experimentally demonstrated in the Davisson–Germer experiment using electrons, and it has also been confirmed for other elementary particles, neutral atoms and even molecules. The wave-like behaviour of matter is crucial to the modern theory of atomic structure and particle physics.
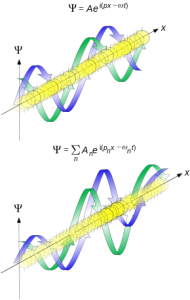
The Wave Properties of Matter:
The fact that light (electromagnetic radiation) exhibited properties of particles became clear from the Compton scattering experiments where the momentum of p = h/λ had to be associated with the x-rays to explain the experimental observations. In 1924 Louis de Broglie proposed that if light waves exhibited properties of particles, then matter particles should exhibit properties of waves, and the wavelength of these waves should be given by the same equation,
Since the wave vector k is defined as k=2πλ, we can rewrite this equation as
Now using the wavelength-momentum relationship λ=ℏp to replace λ we get
Since rp equals the angular momentum, we have
By saying the electron has the property of a standing wave around the orbit, we are led to the conclusion that angular momentum of the electron is quantized in units of ħ. The assumption of quantization thereby is replaced by the postulate that particles have wave properties characterized by a wavelength λ=ℏp, and quantization is a consequence of this new postulate.
Following are a few properties of matter waves:
- Matter waves are not electromagnetic in nature
- Matter-wave represents the probability of finding a particle in space.
- Matter waves or de-Broglie is independent of the charge on the material particle. It means matter-wave of the de-Broglie wave is associated with every moving particle (whether charged or uncharged).
- Electron microscope works on the basis of de-Broglie waves.
- Matter waves can propagate in a vacuum, hence they are not mechanical wave.
- The number of de-Broglie waves associated with the nth orbital electron is n.
- The phase velocity of matter waves can be greater than the speed of light.
- The number of de-Broglie waves associated with the nth orbital electron is n.
- Practical observation of matter waves is possible only when the de-Broglie wavelength is of the order of the size of the particles.
- Only those circular orbits around the nucleus are stable whose circumference is an integral multiple of de-Broglie wavelength associated with the orbital electron.

